How To Calculate Volume Of Gas From Moles

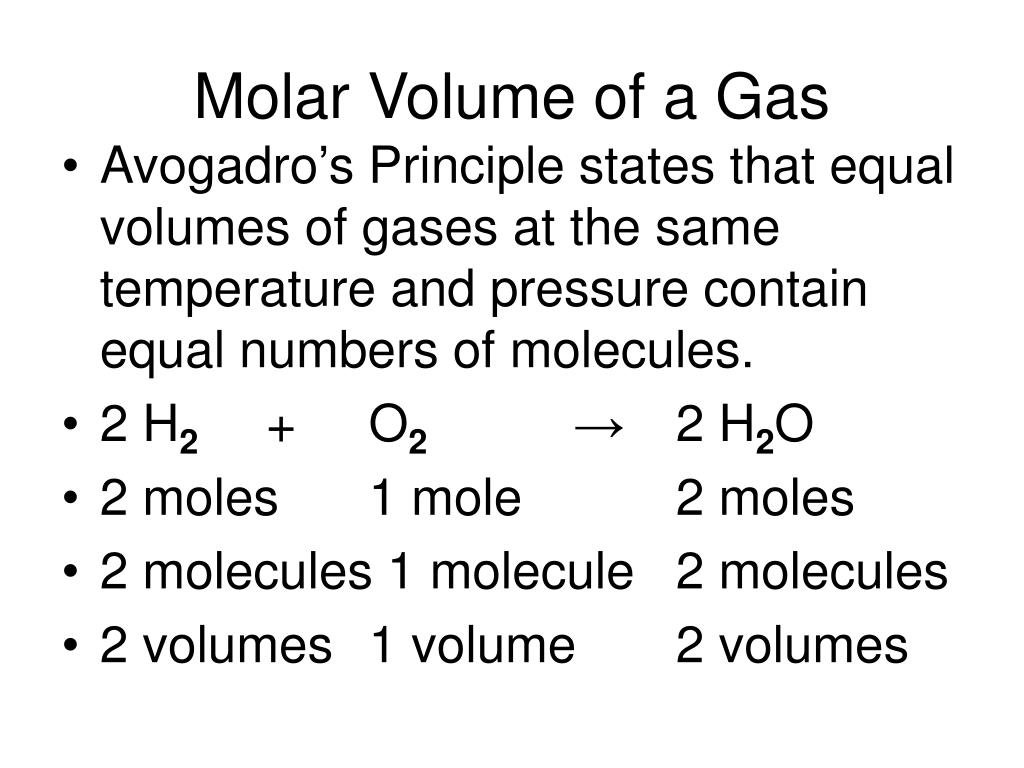
This is Avogadro's law, which states that under the same temperature and pressure, equal volumes of all gases contain the same number of molecules. This equation shows that if the quantity of gas increases, the volume of gas increases proportionally. In other words, the number of gas atoms or molecules is independent of their sizes or the molar mass of the gas.

The law is named after an Italian scientist Amedeo Avogadro who published his hypothesis about the relationship between the gas volume and its amount in moles in 1811. Using this calculator, you can calculate the molar volume of a gas for arbitrary temperature and pressure. Just note that for big values real gases divert from ideal gas law (that's why they are not "ideal") and this formula can't be used. Eventually, these individual laws were combined into a single equation—the ideal gas law—that relates gas quantities for gases and is quite accurate for low pressures and moderate temperatures. We will consider the key developments in individual relationships , then put them together in the ideal gas law. The behavior of gases can be described by several laws based on experimental observations of their properties.

The pressure of a given amount of gas is directly proportional to its absolute temperature, provided that the volume does not change (Amontons's law). The volume of a given gas sample is directly proportional to its absolute temperature at constant pressure (Charles's law). The volume of a given amount of gas is inversely proportional to its pressure when temperature is held constant (Boyle's law). Under the same conditions of temperature and pressure, equal volumes of all gases contain the same number of molecules (Avogadro's law). Gases whose properties of P, V, and T are accurately described by the ideal gas law are said to exhibit ideal behavior or to approximate the traits of an ideal gas.

An ideal gas is a hypothetical construct that may be used along with kinetic molecular theory to effectively explain the gas laws as will be described in a later module of this chapter. Although all the calculations presented in this module assume ideal behavior, this assumption is only reasonable for gases under conditions of relatively low pressure and high temperature. In the final module of this chapter, a modified gas law will be introduced that accounts for the non-ideal behavior observed for many gases at relatively high pressures and low temperatures. One mole of any compound measured in grams is numerically equal to the average mass of one molecule of the compound, in unified atomic mass units. For example, one mole of oxygen with an atomic mass of 16 corresponds to 16 grams. One mole of an ideal gas at standard conditions occupies 22.4 liters.

And is a proportionality constant that relates the values of pressure, volume, amount, and temperature of a gas sample. The variables in this equation do not have the subscripts i and f to indicate an initial condition and a final condition. The ideal gas law relates the four independent properties of a gas under any conditions. Hydrogen gas has the chemical formula H2 and the molecular weight of 2. This gas is the lightest substance among all chemical compounds and the most abundant element in the universe.

Hydrogen gas has also drawn significant attention as a potential energy source. Hydrogen can be obtained, for example, by electrolysis of the water. You calculate the amount of hydrogen in moles either from the gas mass or using the ideal gas law. The ideal gas law formula states that pressure multiplied by volume is equal to moles times the universal gas constant times temperature. As per Avagadro law, equal volume of gases at same temperature and pressure contain equal number of moles regardless of chemical nature of gases.

However Avagadro law is valid only for ideal gases and not for real gases. The ideal gas law is written for ideal or perfect gases. You can use values for real gases so long as they act like ideal gases. To use the formula for a real gas, it must be at low pressure and low temperature. Increasing pressure or temperature raises the kinetic energy of the gas and forces the molecules to interact. While the ideal gas law can still offer an approximation under these conditions, it becomes less accurate when molecules are close together and excited.

Well, if we assume that the temperature and pressure are held constant, then we can substitute our values into the equation. 10 L is our initial volume and 20 L is our final volume. 0.5 moles is our initial quantity, and solving for n2 gives us 1.0 moles.

So, we would need to add 0.5 moles of helium to the balloon to double its volume. Conversion of natural gas volume to weight requires the volume of gas in standard cubic feet and the molecular weight of the natural gas. Is the volume occupied by one mole of a chemical element or a chemical compound. It can be calculated by dividing the molar mass by mass density (ρ). Molar gas volume is one mole of any gas at a specific temperature and pressure has a fixed volume. The volume and temperature are linearly related for 1 mole of methane gas at a constant pressure of 1 atm.

If the temperature is in kelvin, volume and temperature are directly proportional. Charles's law states that the volume of a given amount of gas is directly proportional to its temperature on the kelvin scale when the pressure is held constant. This relationship between temperature and pressure is observed for any sample of gas confined to a constant volume. An example of experimental pressure-temperature data is shown for a sample of air under these conditions in .

To calculate, select the unknown value and enter the known three of the four available values . Click the Calculate button to calculate the unknown value. You can enter the amount in moles or as a combination of the molar mass and the mass of a gas. Use our Molar mass calculator to determine the molar mass of any gas. If you want to determine the molar mass of a mixture of gases such as dry air, you have to determine the molar masses of each gas and multiply them by the mole fractions of each gas. Where Z is the gas compressibility factor, which is a useful thermodynamic property for modifying the ideal gas law to account for behavior of real gases.

The above equation is basically a simple equation of state . If themolecular massof a gas is known, the ideal gas law can be manipulated to find the density of the gas. It's just a matter of plugging in the right variables and performing a few calculations. This ideal gas law calculator determines one of the four values in the ideal gas equation if three others are known. If have 1000 SCF of a natural gas, it is based on the natural gas at standard conditions of 60°F and 14.7 psia – even if the actual temperature and pressure of the gas produced was higher.

If you have actual conditions of pressure and temperature, a conversion from actual gas temperature and pressure is needed to convert the gas volume to standard conditions. To apply this gas law, the amount of gas should remain constant. As with the other gas laws, the temperature must be expressed in kelvins, and the units on the similar quantities should be the same. Because of the dependence on three quantities at the same time, it is difficult to tell in advance what will happen to one property of a gas sample as two other properties change.

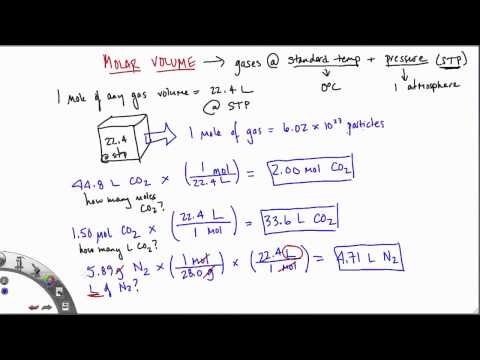
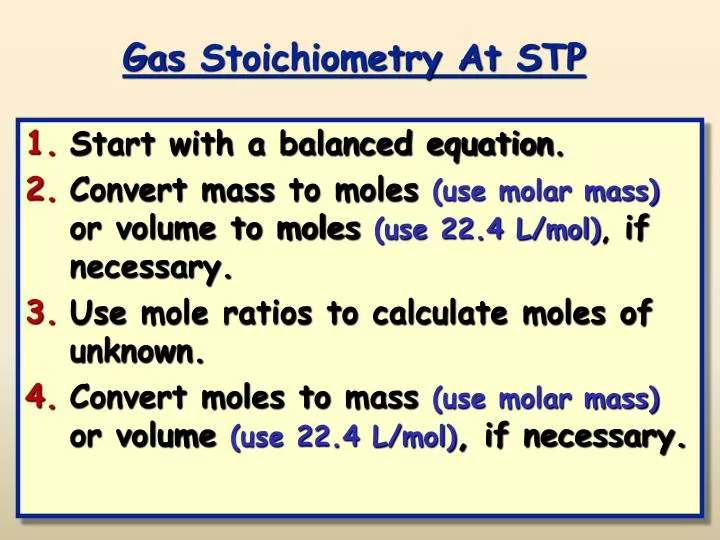
The best way to know is to work it out mathematically. If a sample of gas has an initial pressure of 1.56 atm and an initial volume of 7.02 L, what is the final volume if the pressure is changed to 1,775 torr? Assume that the amount and the temperature of the gas remain constant. Examples and practice problems of solving equation stoichiometry questions with gases. We calculate moles with 22.4 L at STP, and use molar mass and mole ratios to figure out how many products or reactants we have.

The ideal gas equation contains five terms, the gas constant R and the variable properties P, V, n, and T. Specifying any four of these terms will permit use of the ideal gas law to calculate the fifth term as demonstrated in the following example exercises. Molar volume of gases One mole of gas occupies \(\text\) \(\text$\) at standard temperature and pressure. Gases aren't very dense compared to solids and liquids, but they still have a density. Density is defined as mass per volume, and the relation between mass and moles of course is the molecular weight, Mw.

The reader may have noticed that in the examples above, we really didn't care which ideal gas we talked about, just that it was ideal. Usually with density calculations, we need to know what gas we are talking about so we can calculate its molecular weight and thus put mass into the calculation. This is Boyle's law, which describes the relationship between volume V and pressure P of a fixed amount of gas in moles n at a constant temperature T. When the volume and pressure changes, the change of pressure is inversely proportional to the change of volume of the gas. It was formulated by an Anglo-Irish chemist and physicist Robert Boyle in 1662. In Russia and continental Europe, this law is called Boyle's–Mariotte law in recognition of a French physicist and priest Edme Mariotte's contribution to the discovery of this law.

If a sample of gas has an initial pressure of 375 torr and an initial volume of 7.02 L, what is the final pressure if the volume is changed to 4,577 mL? Assume that amount and the temperature of the gas remain constant. One mole of any gas occupies the same volume when measured under the same conditions of temperature and pressure. In this experiment, the volume of one mole of hydrogen is calculated at room temperature and pressure. The interest stems from that accurate measurements of the unit cell volume, atomic weight and mass density of a pure crystalline solid provide a direct determination of the Avogadro constant. The most common molar volume is the molar volume of an ideal gas at standard temperature and pressure (273 K and 1.00 atm).

Multiply the volume and pressure and divide the product by the temperature and the molar gas constant to calculate moles of the hydrogen gas. This means equal amounts of moles of gases occupy the same volume under the same conditions of temperature and pressure. The formula for mass density can be derived from the number density formula by simply multiplying by the molar mass of the gas (shown as just M with units of g/mol). Remember that moles times molar mass is equal to mass . As per Avogadro's law, equal volumes of all gases contain equal number of molecules, at a constant temperature and pressure.

Therefore, equal number of molecules of any gas, must occupy the same volume, at constant temperature and pressure. R is the gas constant also called the ideal, molar, or universal gas constant is a physical constant of proportionality of the ideal gas equation. We can use the ideal gas equation to calculate the volume of 1 mole of an ideal gas at 0°C and 1 atmosphere pressure. Read on to find out more about the ideal gas law, moles, and examples & tips on how to resolve chemical equations. This relationship shows us that if we increase the moles of gas, n, by adding more gas while maintaining the same temperature and pressure, the volume of gas, V, will also increase. The major issue with the idea gas law is that it neglects both molecular size and inter-molecular attractions, therefore it is most accurate for monatomic gases at high temperatures and low pressures.

With lower densities the neglect of molecular size becomes less critical since the average distance between adjacent molecules becomes much larger relative to the size of the molecules themselves. Increasing temperature means higher thermal kinetic energy which diminishes the relative importance of intermolecular attractions. For a constant volume and amount of air, the pressure and temperature are directly proportional, provided the temperature is in kelvin. Imagine filling a rigid container attached to a pressure gauge with gas and then sealing the container so that no gas may escape.

If the container is cooled, the gas inside likewise gets colder and its pressure is observed to decrease. Since the container is rigid and tightly sealed, both the volume and number of moles of gas remain constant. If we heat the sphere, the gas inside gets hotter () and the pressure increases. The ideal gas constant was discovered and introduced in the ideal gas law instead of many specific gas constants by Dmitri Mendeleev in 1877. Therefore, the ideal gas law equation is sometimes, especially in Russian language books, called the Mendeleev-Clapeyron equation. If a sample of gas has an initial pressure of 3.66 atm and an initial volume of 11.8 L, what is the final pressure if the volume is reduced to 5.09 L?

If a sample of gas has an initial pressure of 1.56 atm and an initial volume of 7.02 L, what is the final volume if the pressure is reduced to 0.987 atm? Experience has shown that several properties of a gas can be related to each other under certain conditions. The properties are pressure , volume , temperature , and amount of material expressed in moles . What we find is that a sample of gas cannot have any random values for these properties. Instead, only certain values, dictated by some simple mathematical relationships, will occur. Where x i is the mole fraction of the ith component, M i is the molecular mass of the ith component and ρmixture is the mixture density at the given temperature and pressure.

If we heat the sphere, the gas inside gets hotter and the pressure increases. Use the mass of the hydrogen gas to calculate the gas moles directly; divide the hydrogen weight by its molar mass of 2 g/mole. A balloon contains 0.50 moles of pure helium gas at standard temperature and pressure. Likewise, the only way to decrease the volume of gas, V, while maintaining the same temperature and pressure, is to decrease the moles of gas, n, that are present, that is, remove some of the gas. Likewise, if we decrease the moles of gas, n, by removing some of the gas while maintaining the same temperature and pressure, the volume of gas, V, will also decrease. One major benefit of the behavior of gases is that the volume of one ideal gas in a mixture of ideal gases is equivalent to its mole fraction.

For all practical purposes, the volume fractions and the mole fractions of the components of an ideal gas mixture are interchangeable. For a more precise equation of state you might want to use the van der Waals equation calculator instead of the ideal gas law calculator above. The appropriate formula from the ones listed above is chosen automatically when you use this ideal gas law calculator. The line stops at 111 K because methane liquefies at this temperature; when extrapolated, it intersects the graph's origin, representing a temperature of absolute zero. Note that historically, the empirical gas laws described below led to the derivation of the ideal gas law. These laws were discovered by several scientists who conducted experiments that changed only two state variables of the gas and kept two other variables constant.

The volume-volume problems are the easiest since according to the Law of Combining Gas Volumes, gases combine at the same temperature and pressure in simple whole number of volumes. 1 mole of any gas at STP occupies 22.4 liters of volume. Using this information, the volume occupied by any number of moles can be determined.

Conversely, the number of moles present in any volume of gas at STP can also be determined, and if the identity of the gas is known, this number of moles can be converted to a mass. If a gas has an initial pressure of 24,650 Pa and an initial volume of 376 mL, what is the final volume if the pressure of the gas is changed to 775 torr? A sample of nitrogen gas is confined to a balloon that has a volume of 1.88 L and a pressure of 1.334 atm. What will be the volume of the balloon if the pressure is changed to 0.662 atm?

Assume that the temperature and the amount of the gas remain constant. A gas sample at 35°C has an initial volume of 5.06 L. What is its volume if the temperature is changed to −35°C? Assume that the pressure and the amount of the gas remain constant. A gas sample at 20°C has an initial volume of 20.0 L.

What is its volume if the temperature is changed to 60°C? Chemistry in society Getting the most from reactantsThe molar volume (litres mol-1) is the volume occupied by one mole of any gas at a certain temperature and pressure. The molar volume is the same for all gases at the same temperature and pressure. Gases, involved in chemical reactions show simple ratios between gas volumes.

This makes dealing with gaseous reactions very simple in terms of calculations. When stating molar volume numerical values, it is important to also state the given conditions of temperature and pressure. However, molar volumes are often expressed as cubic metres per 1,000 moles (m3/kmol) or cubic decimetres per mol (dm3/mol) for gases and as centimetres per mole (cm3/mol) for liquids and solids. This online calculator calculates the molar volume of an ideal gas at different conditions .

You can read about the formula and the most commonly used conditions below the calculator. Temperature is sometimes measured with a gas thermometer by observing the change in the volume of the gas as the temperature changes at constant pressure. The hydrogen in a particular hydrogen gas thermometer has a volume of 150.0 cm3 when immersed in a mixture of ice and water (0.00 °C). When immersed in boiling liquid ammonia, the volume of the hydrogen, at the same pressure, is 131.7 cm3. Find the temperature of boiling ammonia on the kelvin and Celsius scales. This calculator allows you to input the data you have in any order you wish - so don't stress if you know the molar mass but not the pressure!